Normal distribution, also known as the Gaussian distribution, is a key concept in statistics that is widely used in various fields, including medicine. At StatisMed, we understand the importance of analyzing data accurately, especially when it comes to medical research. In this blog post, we will delve into the characteristics of normal distribution and how they play a crucial role in statistical analysis.
Understanding Normal Distribution
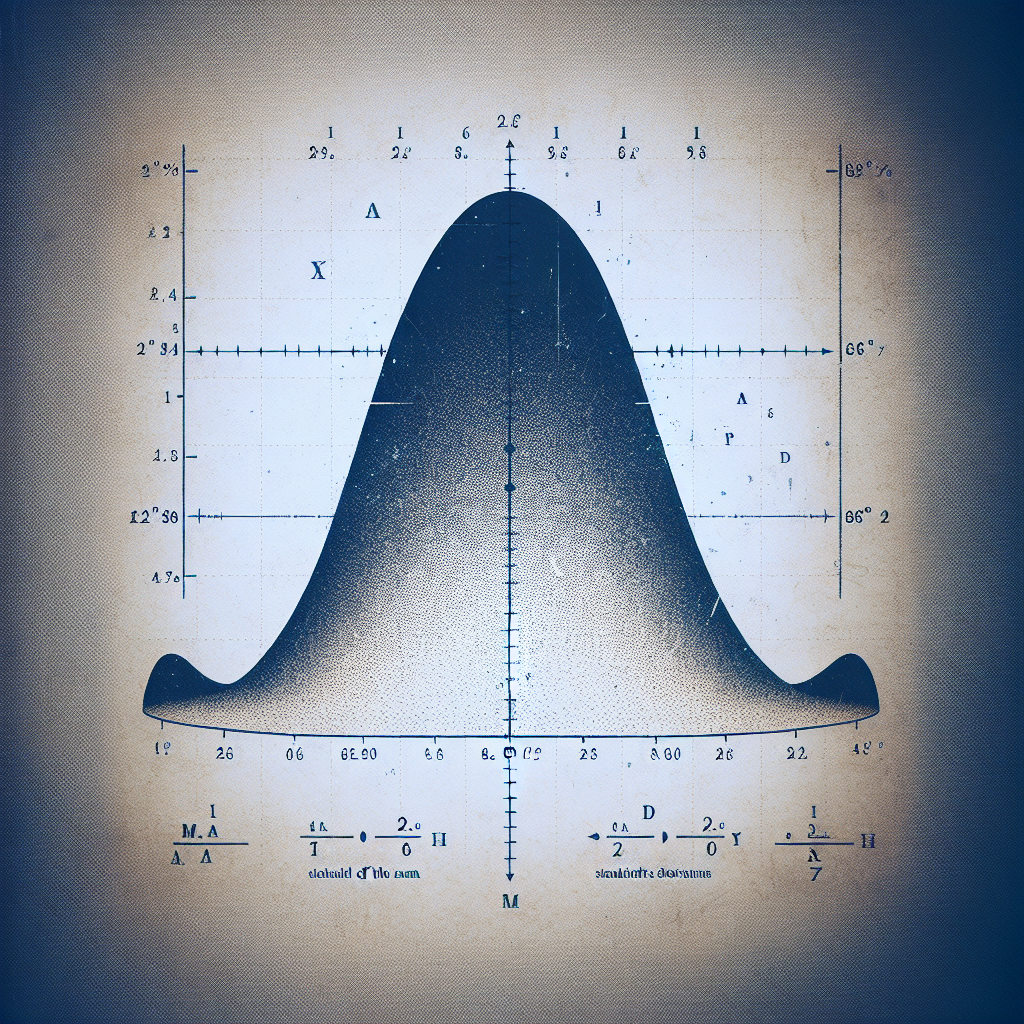
Normal distribution is a bell-shaped curve that is symmetrical around the mean. It is characterized by two parameters: the mean and the standard deviation. The mean represents the average value of the data, while the standard deviation measures the spread of the data points around the mean. In a normal distribution, the majority of the data points fall close to the mean, with fewer data points lying further away.
Key Characteristics of Normal Distribution
-
Symmetry: Normal distribution is symmetric around the mean, with an equal number of data points on either side of the curve.
-
Bell Shape: The curve of a normal distribution is bell-shaped, with the highest point at the mean.
-
68-95-99.7 Rule: This rule states that approximately 68% of the data falls within one standard deviation of the mean, 95% falls within two standard deviations, and 99.7% falls within three standard deviations.
- Empirical Rule: The empirical rule is another way of interpreting the 68-95-99.7 rule, stating that for a normal distribution, approximately 68% of the data falls within one standard deviation of the mean, 95% within two standard deviations, and 99.7% within three standard deviations.
Applications of Normal Distribution in Medicine
Normal distribution is widely used in medical research and clinical practice. It helps in:
-
Diagnosis: Understanding the normal distribution of vital signs and laboratory values helps in diagnosing various conditions.
-
Clinical Trials: Analyzing data using normal distribution helps in determining the efficacy of new treatments.
- Epidemiological Studies: Normal distribution is used to analyze data on disease prevalence and risk factors.
Importance of Normal Distribution in Statistical Analysis
Normal distribution is essential in statistical analysis for the following reasons:
-
Hypothesis Testing: Many statistical tests assume that the data follows a normal distribution, making it crucial for accurate hypothesis testing.
-
Data Modeling: Normal distribution is often used as a model for various phenomena in statistics.
- Predictive Analysis: Understanding the characteristics of normal distribution helps in making accurate predictions based on data.
Conclusion
In conclusion, normal distribution is a fundamental concept in statistics that underpins many analytical techniques used in medical research. Understanding its key characteristics is vital for accurate data analysis and interpretation. At StatisMed, we specialize in providing statistical analysis services for medical professionals, ensuring reliable and insightful results. To learn more about our services, please visit our services page or contact us for a quote.
[ad_2]