Normal distribution, also known as the Gaussian distribution, is a fundamental concept in statistics that plays a crucial role in various fields, including medical research and analysis. At StatisMed, we understand the significance of normal distribution in providing accurate and reliable statistical analysis services for medical professionals. In this blog post, we will delve into the basics of normal distribution, its characteristics, and its importance in statistical analysis.
What is Normal Distribution?
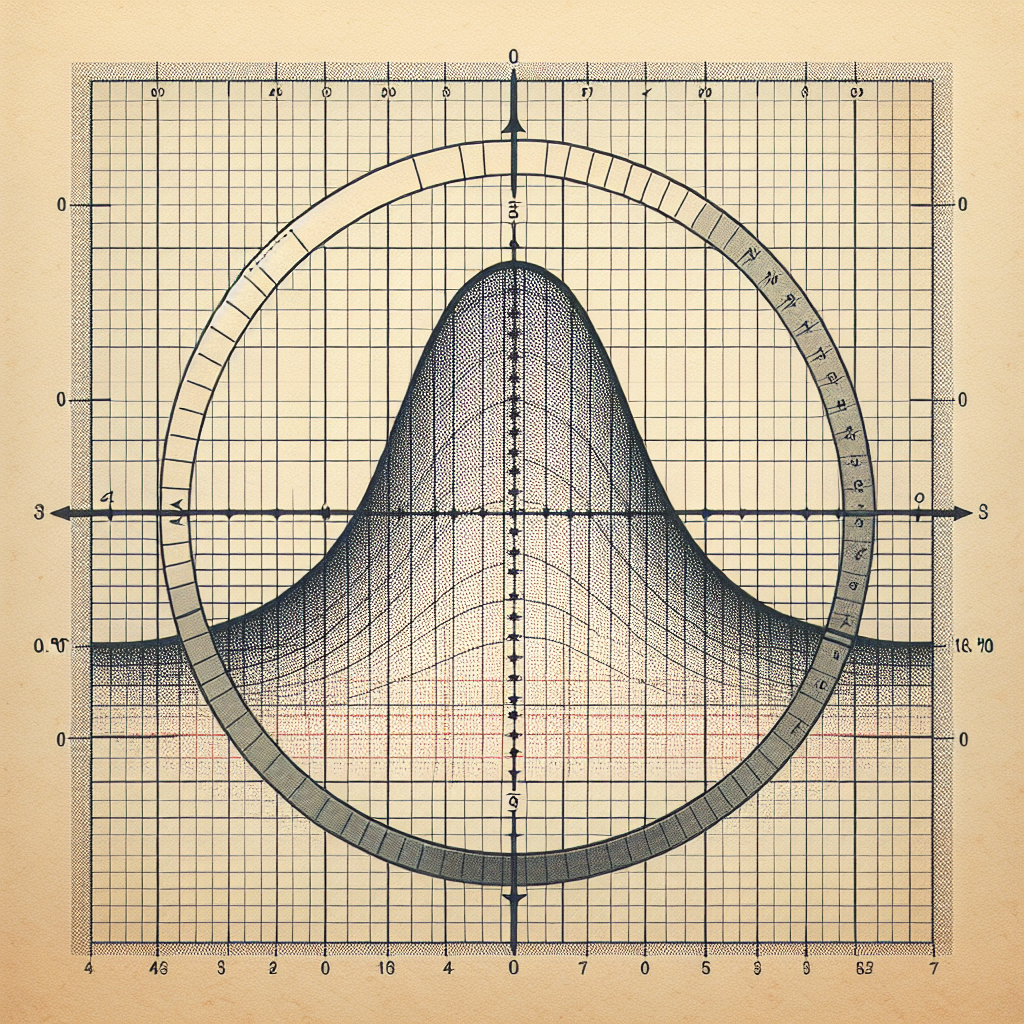
Normal distribution is a bell-shaped probability distribution that is symmetric around its mean. It is characterized by a smooth, symmetric curve that represents the probability distribution of a continuous random variable. In a normal distribution, the mean, median, and mode are all equal, and their values lie at the center of the distribution. The distribution is defined by two parameters: the mean (μ) and the standard deviation (σ).
Characteristics of Normal Distribution
- Symmetry: The normal distribution in statistics is symmetric around its mean, with half of the values falling to the left and half to the right of the mean.
- Bell-shaped curve: The curve of the normal distribution is bell-shaped, with the highest point at the mean.
- Mean, median, and mode: In a normal distribution, the mean, median, and mode are all equal and located at the center of the distribution.
- Standard deviation: The spread of data in a normal distribution is determined by the standard deviation, which measures the variation of data points from the mean.
Importance of Normal Distribution in Statistics
Normal distribution is essential in statistics for several reasons:
- Statistical Inference: Normal distribution serves as the basis for many statistical tests and techniques, such as hypothesis testing and confidence intervals.
- Population Studies: Many natural phenomena, such as human height and IQ scores, follow a normal distribution, making it valuable for population studies and analysis.
- Predictive Modeling: In predictive modeling and regression analysis, assuming a normal distribution can help in making accurate predictions and inferences.
Applications of Normal Distribution in statistics in Medical Research
Normal distribution is widely used in medical research and analysis for various purposes, including:
- Clinical Trials: Normal distribution is used to analyze clinical trial data and determine the effectiveness of medical treatments.
- Epidemiological Studies: Normal distribution helps in studying the distribution of diseases in populations and predicting disease trends.
- Healthcare Data Analysis: Normal distribution enables healthcare professionals to analyze patient data, identify trends, and make informed decisions.
Understanding Normal Distribution Parameters
In a normal distribution, the mean (μ) represents the central tendency of the data, while the standard deviation (σ) measures the dispersion of data points around the mean. The empirical rule, also known as the 68-95-99.7 rule, states that within one standard deviation of the mean, approximately 68% of the data falls. Within two standard deviations, about 95% of the data falls, and within three standard deviations, around 99.7% of the data falls 1.
Conclusion
In conclusion, normal distribution is a foundational concept in statistics that underpins many statistical analyses and techniques. At StatisMed, we leverage the principles of normal distribution to provide accurate and reliable statistical analysis services for medical professionals. By understanding the basics of normal distribution and its applications in medical research, healthcare professionals can make informed decisions and advancements in the field of medicine.
For more information on our statistical analysis services, please visit our Services page. If you’d like to learn more about our company, visit our About Us page. Feel free to contact us or request a quote for any statistical analysis needs.
Remember, understanding normal distribution is key to unlocking valuable insights in medical research and statistical analysis.
[ad_2]